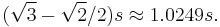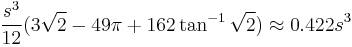Reuleaux tetrahedron
The Reuleaux tetrahedron is the intersection of four spheres of radius s centered at the vertices of a regular tetrahedron with side length s. The sphere through each vertex passes through the other three vertices, which also form vertices of the Reuleaux tetrahedron. The Reuleaux tetrahedron has the same face structure as a regular tetrahedron, but with curved faces: four vertices, and four curved faces, connected by six circular-arc edges.
This shape is defined and named by analogy to the Reuleaux triangle, a two-dimensional curve of constant width. One can find repeated claims in the mathematical literature that the Reuleaux tetrahedron is analogously a surface of constant width, but it is not true: the two midpoints of opposite edge arcs are separated by a larger distance,
The volume of a Reuleaux tetrahedron is [1]
Meissner bodies
Meissner and Schilling[2] showed how to modify the Reuleaux tetrahedron to form a surface of constant width, by replacing three of its edge arcs by curved patches formed as the surfaces of rotation of a circular arc. According to which three edge arcs are replaced (three that have a common vertex or three that form a triangle) there result two noncongruent shapes that are sometimes called Meissner bodies or Meissner tetrahedra (pictures and films in Weber 2009[3]). Bonnesen and Fenchel[4] conjectured that Meissner tetrahedra are the minimum-volume three-dimensional shapes of constant width, a conjecture which is still open[5]. In connection with this problem, Campi, Colesanti and Gronchi[6] showed that the minimum volume surface of revolution with constant width is the surface of revolution of a Reuleaux triangle through one of its symmetry axes.
References
- ^ Weisstein, Eric W (2008), Reuleaux Tetrahedron, MathWorld–A Wolfram Web Resource, http://mathworld.wolfram.com/ReuleauxTetrahedron.html
- ^ Meissner, Ernst; Schilling, Friedrich (1912), "Drei Gipsmodelle von Flächen konstanter Breite", Z. Math. Phys. 60: 92–94
- ^ Weber, Christof (2009). "What does this solid have to do with a ball?". http://www.swisseduc.ch/mathematik/material/gleichdick/docs/meissner_en.pdf. There are also films of both types of Meissner body rotating.
- ^ Bonnesen, Tommy; Fenchel, Werner (1934), Theorie der konvexen Körper, Springer-Verlag, pp. 127–139
- ^ Kawohl, Bernd; Weber, Christof (2011), "Meissner's Mysterious Bodies", Math. Intell. 33: Nr. 3, 94–101
- ^ Campi, Stefano; Colesanti, Andrea; Gronchi, Paolo (1996), "Minimum problems for volumes of convex bodies", Partial Differential Equations and Applications: Collected Papers in Honor of Carlo Pucci, Lecture Notes in Pure and Applied Mathematics, no. 177, Marcel Dekker, pp. 43–55
External links
- Lachand-Robert, T. & Oudet, É.. "Spheroforms". http://www.lama.univ-savoie.fr/~lachand/Spheroforms.html.